Intended Audience: Teachers, prospective teachers, and parents (public, private, homeschool).
In this video, Autumn and I describe the number Pi and say a little bit about the history of one of the most famous numbers. Happy Pi day!
Continue readingMy 2018 NCTM Presentation: Story Archetypes in Mathematics Curricula
Are you going to the NCTM 2018 conference next week in Washington, DC? Do you want to learn about the thinking behind the Eureka Math/EngageNY curriculum from the lead writer and lead mathematician? Then put the following talk on your schedule:
Story Archetypes in Mathematics Curricula: How is Eureka Math/EngageNY like STAR WARS?
Scott Baldridge
Thursday, 26 April, 2018
3:00 PM – 4:00 PM
Walter E. Washington Convention Center
Room: Ballroom C
Ken Ono: Gems of Ramanujan and their Lasting Impact on Mathematics, Lecture 1
Intended Audience: Everyone: Students, teachers, prospective teachers, and parents.
Ramanujan’s work has had a truly transformative effect on modern mathematics, and continues to do so as we understand further lines from his letters and notebooks. In this YouTube video, Ken Ono presents some of the studies of Ramanujan that are most accessible to the general public and talks about how Ramanujan’s findings fundamentally changed modern mathematics. Continue reading
You can use pictures to explain column addition!
Intended Audience: Teachers, prospective teachers, and parents (public, private, homeschool).
In this video, 7-year-old Autumn explains the addition algorithm (column addition) using place value tables. We address the story of a frustrated dad who wrote a “place value table” on a check and how his frustration is not with place value tables. We discuss good ways to use place value tables to teach the addition algorithm and when place value tables have overstayed their purpose. Enjoy!
More Good News: Middle School Students’ Scores Jump with Eureka Math
Good news just keeps rolling in: Students in Partnership Schools who started using Eureka Math last year showed an 8% improvement in seventh grade and a 10% improvement in eighth grade on the end-of-year New York state math test over the previous year students:
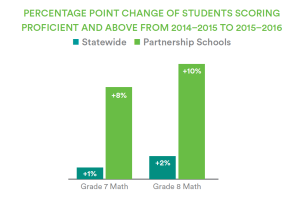Continue reading
Tim Nguyen: The Perturbative Approach to Path Integrals
Intended Audience: Mathematicians, physicists, graduate students, and advanced undergraduates.
In this video, mathematician Tim Nguyen gives an informative overview of the perturbative approach to path integrals and explains his work on the features of path integral manipulations.
Continue reading
Why should a parallelogram also be a trapezoid? The answer may surprise you.
Here is an excellent question from a user of Eureka Math:
I was reviewing the Grade 4 Module 4 lessons and videos and saw the definition of a trapezoid as a quadrilateral with at least one pair of parallel sides. I know this is correct, but my question is, how useful is this vs. a previous common understanding (or misunderstanding) that many of us had, that a trapezoid is a quadrilateral with exactly one pair of parallel sides? I was just thinking about this today and couldn’t think of how being able to classify all parallelograms also as trapezoids is useful, whereas it seemed very clean and clear to identify and recognize quads with only one pair of parallel sides as trapezoids and to be able to say “this is what a trapezoid is” rather than “this is one type of trapezoid”. Any thoughts on this?
A little self-love? Blah…
On the big list of things to do, making a biography of myself is at the very bottom of the priority list. It’s one of those things you eventually get roped into doing for other reasons. I’ve finally created a short bio that you find on my “about page” above or by clicking here. Enjoy?
While you’re at it, subscribe to my YouTube channel because it is the cool thing to do.
CHANNEL: That’s News to Me
© 2016 Scott Baldridge
Learning Division Facts: Numbers that are Products of Two 1-digit Numbers
Intended Audience: Teachers, prospective teachers, and parents (public, private, homeschool).
In this video, 7-year-old Autumn tells whether a number from 1-100 is the product of two 1-digit numbers. This is an important step along the way of using the multiplication table to learn the division facts.
Mathematician Pallavi Dani – Divergence in Right-angled Coxeter Groups
Intended Audience: Everyone, and especially teachers who want to show to their students a mathematician explaining the motivation behind their own research.
In this episode we meet Pallavi Dani, a mathematician here at Louisiana State University, who talks to us about using geometry to study problems in algebra and vice versa.
Posted in Geometry and Topology Today
Tagged Algebra, Coxeter Groups, Divergence Functions, Fundamental Domains, Geometry, Geometry and Topology Today, Group Theory, Hyperbolic Groups, Louisiana State University, LSU, Mathematics Department, Pallavi Dani, Quasi-isometry Invariants, Right-angled Coxeter Groups, Scott Baldridge, Shea Vela-Vick, Topology
2 Comments
But Honey, You do know the Multiplication Table!
Intended Audience: Teachers, prospective teachers, and parents (public, private, homeschool).
In this video, 7-year-old Autumn realizes that she does know the complete multiplication table! Here’s the story:
Fraction Multiplication via Skip Counting!
Intended Audience: Teachers, prospective teachers, and parents (public, private, homeschool).
In this video, 7-year-old Autumn uses skip counting to find 
A Portrait of an Inspiring Teacher: Fred Reusch
Tom Rademacher wrote a nice piece about my stepfather’s teaching:
Fred Reusch: Teacher, Tutor, ‘Coolest Man Ever’
You may remember my dad from one of my earlier posts, What it is like to be the son of Captain Derivative, and his picture:
Here are a couple of my thoughts about Rademacher’s article and my dad that will hopefully add a little “lagniappe” to the piece and Fred’s teaching:
Posted in Commentary, That's News To Me
Tagged Captain Derivative, Differentiation, Fred Reusch, high school, math, mathematics, Scott Baldridge, Teaching
1 Comment
Clayton Shonkwiler: Research Seminar on Applications of Geometry and Topology to Random Walks
Intended Audience: Research mathematicians, graduate students, and advanced undergraduates.
In this episode we see a research talk presented by Clayton Shonkwiler, a mathematician at Colorado State University, who is speaking to us about applications of geometry and topology to random walks/polygons and polymer physics.
Can an atom have two nuclei? An inquisitive child wants to know!
My 7-year-old daughter, Autumn, and me were discussing how an atom is made up of mostly empty space with a small nucleus of protons and neutrons in the “center” and electrons swarming about it. During the discussion, she asked an insightful question,
“Can an atom have two cores (two nuclei)?”
The Washington Post: Many parents hated Common Core math at first, before figuring it out
Columnist Jay Mathews writes in a new Washington Post article, Many parents hated Common Core math at first, before figuring it out,
Dear Mr. Mathews: Thank you for sharing these stories. Continue reading
Mathematician Clayton Shonkwiler: An Advanced Perspective
Intended Audience: Mathematicians, graduate students and ambitious high school students.
We continue our interview with Clayton Shonkwiler on applications of geometry and topology to random walks/polygons and polymer science, but now at a graduate student level. To see the earlier interview with Clayton geared at a high-school level, go to: Mathematician Clayton Shonkwiler talks about Polymer Science.
Growing up with Eureka Live: Mathematical reasons for introducing a different solution method
Intended Audience: Teachers, prospective teachers, and parents (public, private, homeschool).
In this video, 7 year old Autumn leads an audience of about 100 teachers in doing arithmetic problems. Watch her explain how she uses different methods to solve problems other than the “standard column math” method (algorithms), and allow me to explain why it is important to build curricula that encourage all of these methods.
Holiday Math Special: In the 12 Days of Christmas song, which of the presents do you get the most?
Intended Audience: Teachers, prospective teachers, and parents (public, private, homeschool).
Recently Autumn asked, “In the twelve days of Christmas, which of the presents do you get the most?” This is the type of question you hope your child asks you, because it can lead everyone in the family on a great adventure where math just “happens” in the course of thinking through the answer.
Multiplying by 25
Intended Audience: Teachers, prospective teachers, and parents (public, private, homeschool).
Autumn is now 7 years old! Thank you to everyone out there who has been watching her grow up. We have many more great videos planned for this year, so please like us on Facebook (www.fb.com/ScottJBaldridge) or follow us @ScottBaldridge, or follow this blog for updates.
Woman Superhero T-Shirt Challenge
Autumn asked, “Why don’t you wear a girl superhero T-shirt for our videos, dad?” And I said, “Why not? Absolutely!” We started searching for a woman superhero T-shirt for men but ran into trouble: There are a lot of women superhero T-shirts for women, but very few for men that are perfect for our video series. So we need your help!
If you know of an excellent woman superhero T-shirt for men, please share it with us in the comment section below. If you include your name (you don’t have to), we will thank you in the video when I wear your T-shirt!
CHANNEL: Growing up with Eureka
© 2015 Autumn Baldridge and Scott Baldridge
Mathematician Clayton Shonkwiler talks about Polymer Science
Intended Audience: Everyone, and especially teachers who want to show to their students a mathematician explaining the motivation behind their own research.
In this episode we meet Clayton Shonkwiler, a mathematician from Colorado State University, who talks to us about applications of geometry and topology to the study of random polygons and polymer science. Continue reading
Variables made easy
Intended Audience: Teachers, prospective teachers, and parents (public, private, homeschool).
In this video, 6-year-old Autumn explains that a variable is a slot that you can put a number into. The slot is usually represented on paper as a letter (such as x) or a mark (such as ___). Here’s the definition of a variable symbol: Continue reading
Building the ⟨Sci|State⟩ Studio—a weekend in time lapse.
The SciState Studio is finished and we’re all ready for production! In this video, we get a behind the scenes look at some of the finer details of our studio construction. As we embarked on this adventure, one of the first things we learned was that high-quality audio is an essential component of any successful video series. With this in mind, we have outfitted our studio with acoustic insulation panels and installed various other echo reducing components throughout. We also constructed an awesome plexiglass “light board,” which is similar to a traditional whiteboard but doesn’t require one’s back to face the camera. We’re really proud of everything we’ve produced and hope you agree!
We should point out that it takes a real expert (thank you Justin Reusch!) to set up the lighting for the video stage. You can see how that came out by watching our “Testing out the Green Screen” video. As a point of comparison, note the terrible sound in the “Testing out the Green Screen” video—that is what the studio sounded like before we built the acoustic insulation panels.
As always, comments are welcome below.
CHANNEL: Geometry and Topology Today
© 2015 Scott Baldridge and David Shea Vela-Vick
Supported by NSF CAREER grant DMS-0748636 and NSF grant DMS-1249708
Something fun: What it is like to be the son of Captain Derivative
Above is a picture of my stepdad, Captain Derivative, doing integration exercises for yet another day in the vector field against his arc enemy, the pathological and degenerate Prime Matrix.
Some people have asked me, “What is it like being the son of a differential operator who can wield power series with such ease?” Well, when I was young and still very near my initial value, I used to oscillate rapidly between believing whether his exponential powers were real or imaginary–often shifting my phase over time with some frequency. But then he removed a discontinuity from a complex surface right before my very eyes, and I saw the proof of his limitless analysis: I converged upon the realization that my own scientific skepticism had been irrational. From that moment on (t=5), I would often help him from his secret power base by relaying coordinates of tangents he could approach in the Cartesian plane so he could do battle against those divergent improper integrals.
Go Captain Derivative, we are with you (or at least, within an epsilon ball of you). May the functions you differentiate always be smooth!
(My stepfather, Fred Reusch, is the calculus teacher at my old high school in Rockford, Michigan. Today was “Super-hero Day” at the school, and he came dressed as “Captain Derivative.” I think he would wear this outfit to school everyday if he could! 🙂 )
CHANNEL: That’s News To Me
© 2015 Scott Baldridge
Posted in That's News To Me
Tagged Captain Derivative, Differentiation, Fred Reusch, Scott Baldridge
1 Comment
Adam Saltz speaks on an annular refinement of the transverse element in Khovanov homology
Intended Audience: Research mathematicians, professors of mathematics, graduate students in mathematics, and advanced undergraduate students in mathematics.
In this 1 hour episode, we see a presentation by Adam Saltz, a mathematician and graduate student at Boston College, on a new invariant of transverse knots in links coming from Khovanov homology.
In the talk, Adam discusses some of the details contained in his paper with Diana Hubbard, An annular refinement of the transverse element in Khovanov homology. Here is the abstract to their paper:
We construct a braid conjugacy class invariant κ by refining Plamenevskaya’s transverse element ψ in Khovanov homology via the annular grading. While κ is not an invariant of transverse links, it distinguishes some braids whose closures share the same classical invariants but are not transversely isotopic. Using κ we construct an obstruction to negative destabilization (stronger than ψ) and a solution to the word problem in braid groups. Also, κ is a lower bound on the length of the spectral sequence from annular Khovanov homology to Khovanov homology, and we obtain concrete examples in which this spectral sequence does not collapse immediately. In addition, we study these constructions in reduced Khovanov homology and illustrate that the two reduced versions are fundamentally different with respect to the annular filtration.
This video and paper are aimed at mathematicians, graduate students and undergraduates with lots of experience in topology. However, high school students who think they are potential math geniuses may still enjoy looking at it to see what advanced theorems and proofs look like.
As always, comments are welcome!
CHANNEL: Geometry and Topology Today
© 2015 Scott Baldridge and David Shea Vela-Vick
Supported by NSF CAREER grant DMS-0748636 and NSF grant DMS-1249708
Testing out the new Green Screen (Plus a bonus 14×16 calculation)
This is a test video that Shea and I did to test the new green screen. Shea is spoofing a bit with me—acting all surprise at my ability to calculate 14×16 in my head. He looses it slightly near the end. (Research mathematicians do a lot more than mental math although we are almost constantly doing mental math: it is just math that is a lot harder than simple arithmetic.) Check out Shea laughing at the end.
The test video is to try the different types of backgrounds we can now use with the new studio setup (and green screen). You can vote which background you like the most here:
This is just an informal poll. You can comment below if you have a better idea for a background. For example, Shea’s son wanted whales swimming. Maybe not quite professional enough for math videos…
Thanks especially goes to Justin Reusch, who came all the way from Austin, Texas to set up—and explain how to set up—the lighting for this type of shoot. You can see his work in the video on our faces (the back-light halo effect on our heads, the side lights and shadows on our faces, etc. ). Thank you, Justin! Thanks also goes to Hang, who kept the kids busy while we put together the shot. You can hear the kids in the background playing during the video: like I said, this was just a test video.
CHANNEL: Geometry and Topology Today
© 2015 Scott Baldridge and David Shea Vela-Vick
Supported by NSF CAREER grant DMS-0748636 and NSF grant DMS-1249708
Powers of 2
Intended Audience: Teachers, prospective teachers, and parents (public, private, homeschool).
In this video, 6-year-old Autumn explains that the meaning of “2 to the power of 4” is a product of four factors of 2:
This is the beginning of exponentiation and it is easy to understand—the power (in this case, 4) tells us how many factors of the base (2) there should be. I usually tell my college students tongue-in-cheek that, “Mathematicians are laaaaazzzzzy. We came up with the notation 
There are a few issues to watch out for when introducing a child to exponents (some of which I mentioned in the video):
- At this level, after students understand multiplication, powers of 2 are no harder or easier than learning addition or multiplication facts.
- However, it is a new operation and one that is easily confused with multiplication. The statement, “2 times 4,” sounds a lot like, “2 to the 4th.” Furthermore, the processes for evaluating the expressions are are similar but for powers we use multiplication instead of addition: “2 times 4” is describing a number of addends while “2 to the 4th” is describing a number of factors.
For these reasons, you (as the parent or teacher) should be very deliberate about asking, “What is 2 to the power of 4?” to help your child/students understand that you are asking for something very different than “2 fours.”
- Definitely stick with “powers of 2” until students are comfortable with the meaning of exponents and can confidently tell you what
are. Remember, you are trying to help students learn the meaning of the operation, not memorize a bunch of numbers. (If you feel you can move on, go to powers of 10 next, and then to powers of 3.)
Once they are comfortable moving back and forth between
and
the real fun can begin—learning the properties of exponentiation like
Don’t worry about this for now; Autumn and I will show you how easy and pleasurable it is to learn some of these properties in another video. For now, just concentrate on learning the powers of 2 themselves (up to 10 or 12).
- Finally, it is much easier to remember the powers of 2 if you use “tags” or “pins,” i.e., memorizing a couple of easy-to-remember powers of 2, and using them to quickly figure out the rest. I recommend:
,
, and
. Then one can quickly find
, which is just an easy double. You will see the “story” Autumn and I used to remember
Check back soon for a new video on the properties of exponentiation. In the meantime, enjoy learning the powers of 2 with your child/students!
As always, comments are welcomed.
CHANNEL: Growing up with Eureka
© 2015 Autumn Baldridge and Scott Baldridge
Partially supported by NSF CAREER grant DMS-0748636
An article on how Eureka Math began.
Leigh Guildry wrote a nice piece for The Town Talk newspaper, “How did Eureka Math start? 2 writers answer.” She traces the steps that I, Robin Ramos, Nell McAnnelly, and Lynne Munson (director of Great Minds) took to develop the Eureka Math/Engage NY curriculum.
She starts with recent test results of students from Rapides Parish School System who were using Eureka Math:
“Rapides [Parish School System] students progressed at almost double the national average on benchmark tests by Discovery Education. Students gained an average of 141 scale score points during the 2014–15 school year, compared to 73 points for students in districts nationally, according to eureka-math.org. The district more than doubled the average U.S. gains in middle school grades (6–8) and in kindergarten.”
Pretty impressive! Congratulations go to students and their teachers.
The article goes on to interview Pam Goodner, who was the lead writer who worked with me in creating the 12th grade “Precalculus Course.” (Thank you, Pam, for your hard work.)
Louisiana Bayou, JR Meeker (1884) wiki commons
You can follow Leigh Guidry on twitter at @Leigh_TownTalk.
CHANNEL: That’s News to Me
© 2015 Scott Baldridge
Scott Baldridge 
MSU is Tearing Down Obstacles to Science and Engineering Degrees
Last week I had an opportunity to visit a university that is working hard to improve its beginning math courses: Michigan State University. Continue reading →